Difference between revisions of "Damped oscillation"
Max Peschke (talk | contribs) |
Max Peschke (talk | contribs) |
||
| Line 46: | Line 46: | ||
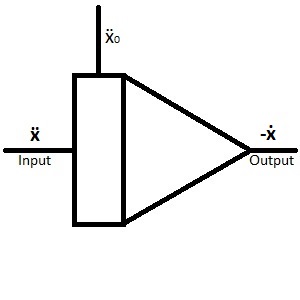
| On the right side you find the basic circuit symbol of an integrator, where the input (or inputs) can be found on the left and the output on the right. Coming from above you find '''ẍ<sub>0</sub>''', which represents the starting condition of the integrator and can be thought of as the integration constant. || [[File:DO Int01.jpg|thumb|300px|Basic Integrator Scheme]] | | On the right side you find the basic circuit symbol of an integrator, where the input (or inputs) can be found on the left and the output on the right. Coming from above you find '''ẍ<sub>0</sub>''', which represents the starting condition of the integrator and can be thought of as the integration constant. || [[File:DO Int01.jpg|thumb|300px|Basic Integrator Scheme]] | ||
|- | |- | ||
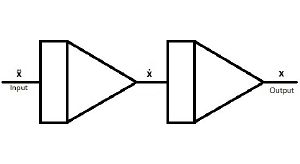
| − | | When putting two integrators together, you can generate '''ẋ''' and '''x''' || [[File:DO Int02.jpg|thumb|300px| | + | | When putting two integrators together, you can generate '''ẋ''' and '''x''' || [[File:DO Int02.jpg|thumb|300px|Generating all derivatives]] |
|- | |- | ||
| − | | | + | | Lala || Example |
|- | |- | ||
| Example || Example | | Example || Example | ||
|} | |} | ||
Revision as of 15:02, 6 December 2021
The modeling of a damped oscillation is a good starting point for analog programming beginners. This article shall give a detailed explanation how to implement a simulation on The Analog Thing using the full repatriation method to derive a computer circuit (originally developed by Lord Kelvin around 1875) and to get results on an oscilloscope.
There are four major steps, all of which are equally crucial:
- Describe the to be simulated system with differential equations
- Derive a computer circuit from these equations
- Wire the computer circuit on the analog computer and adjust parameters
- Choose viable visualization method (usually oscilloscopes) and connect the computer circuit
This article will focus on point 2. to 4. and requires a basic understanding of differential equations.
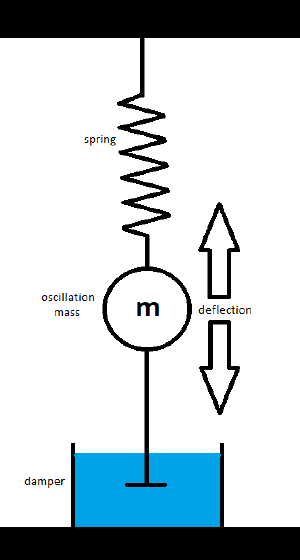
1. Mathematical description of a damped oscillation
The first and often hardest step of modeling a to be simulated system on an analog computer is to give an exact mathematical description of the system in the form of differential equations. For this example the description is rather short:
Find all acting forces, three in this case:
- Spring force Fs = k*x;
k = spring coefficient, x = deflection
- Inertia force Fm = m*a;
m = mass, a = acceleration
- Damper force Fd = d*v;
d = damper coefficient, v = velocity
Set the sum of all forces to zero (definition of an isolated system):
Fm + Fd + Fs = 0 m*a + d*v + k*x = 0
Replace the velocity v with ẋ and the acceleration a with ẍ
v = ẋ
(the velocity equals the first derivative of x)
a = ẍ
(the acceleration equals the second derivative of x)
m*a + d*v + k*x
= m*ẍ + d*ẋ + k*x = 0
Now we have an exact description the damped oscillation in the form of a differential equation.
Lastly, solve this equation for the highest derivative the finish the full repatriation:
-> ẍ = -(d*ẋ + k*x) / m
2. Derivation of a computer circuit
Very often the starting point is an integrator, which will in this case transform ẍ to ẋ.
| Description | Circuit |
|---|---|
| On the right side you find the basic circuit symbol of an integrator, where the input (or inputs) can be found on the left and the output on the right. Coming from above you find ẍ0, which represents the starting condition of the integrator and can be thought of as the integration constant. | |
| When putting two integrators together, you can generate ẋ and x | |
| Lala | Example |
| Example | Example |